Answer:
He must survey 123 adults.
Explanation:
In a sample with a number n of people surveyed with a probability of a success of
 , and a confidence level of
, and a confidence level of
 , we have the following confidence interval of proportions.
, we have the following confidence interval of proportions.

In which
z is the z-score that has a p-value of
 .
.
The margin of error is:

Assume that a recent survey suggests that about 87% of adults have heard of the brand.
This means that
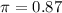
90% confidence level
So
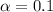 , z is the value of Z that has a p-value of
, z is the value of Z that has a p-value of
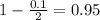 , so
, so
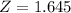 .
.
How many adults must he survey in order to be 90% confident that his estimate is within five percentage points of the true population percentage?
This is n for which M = 0.05. So

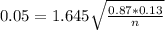
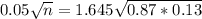
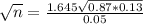
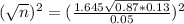
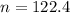
Rounding up:
He must survey 123 adults.