Question

There were 2,300 applicants for enrollment to the freshman class at a small college in the year 2010. The number of applicants has risen linearly by roughly 170 per year. The number of applications f(x) is given by f(x) = 2,300 + 170x, where x is the number of years since 2010. a. Determine if the function g(x) = * = 2,300 is the inverse of f. 170 b. Interpret the meaning of function g in the context of the problem.

a. No
b. The value g(x) represents the number of years since the year 2010 based on the number of applicants to the freshman class, x.
a. Yes
b. The value 8(x) represents the number of applicants to the freshman class based on the number of years since 2010,
a. No
b. The value slx) represents the number of applicants to the freshman class based on the number of years since 2010,
a. Yes
b. The value six) represents the number of years since the year 2010 based on the number of applicants to the freshman class x

Answer 1

The inverse function is
`g(x) = (x - 2300)/(170)`

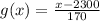
The value of g(x) represents the number of applicants to the freshman class based on the number of years since 2010.

Explanation:

Number of applicants in x years after 2010:

Is given by the following function:

`f(x) = 2300 + 170x`

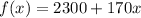
Inverse function:

We exchange the values of y = f(x) and x in the original function, and then find y. So

`x = 2300 + 170y`

`170y = x - 2300`

`y = (x - 2300)/(170)`

`g(x) = (x - 2300)/(170)`

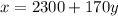
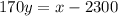
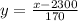
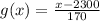
The inverse function is
`g(x) = (x - 2300)/(170)`

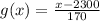
Meaning of g:

f(x): Number of students in x years:

g(x): Inverse of f(x), is the number of years it takes for there to be x applicants, so the answer is:

The value of g(x) represents the number of applicants to the freshman class based on the number of years since 2010.