Question

The number of calls received by an office on Monday morning between 8:00 AM and 9:00 AM has a mean of 5. Calculate the probability of getting at least 4 calls between eight and nine in the morning.

Answer 1

0.735 = 73.5% probability of getting at least 4 calls between eight and nine in the morning.

Explanation:

We have the mean during a time interval, which means that the Poisson distribution is used to solve this question.

In a Poisson distribution, the probability that X represents the number of successes of a random variable is given by the following formula:

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

In which

x is the number of sucesses

e = 2.71828 is the Euler number

`\mu` is the mean in the given interval.

The number of calls received by an office on Monday morning between 8:00 AM and 9:00 AM has a mean of 5.

This means that
`\mu = 5`

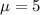
Calculate the probability of getting at least 4 calls between eight and nine in the morning.

This is:

`P(X \geq 4) = 1 - P(X < 4)`

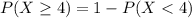
In which

`P(X < 4) = P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3)`

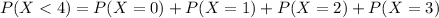
So

`P(X = x) = (e^(-\mu)*\mu^(x))/((x)!)`

`P(X = 0) = (e^(-5)*5^(0))/((0)!) = 0.0067`

`P(X = 1) = (e^(-5)*5^(1))/((1)!) = 0.0337`

`P(X = 2) = (e^(-5)*5^(2))/((2)!) = 0.0842`

`P(X = 3) = (e^(-5)*5^(3))/((3)!) = 0.1404`

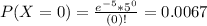
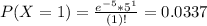
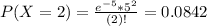
Then

`P(X < 4) = P(X = 0) + P(X = 1) + P(X = 2) + P(X = 3) = 0.0067 + 0.0337 + 0.0842 + 0.1404 = 0.265`

`P(X \geq 4) = 1 - P(X < 4) = 1 - 0.265 = 0.735`

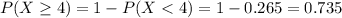
0.735 = 73.5% probability of getting at least 4 calls between eight and nine in the morning.