Answer:
The dimensions are:
l = 2*1.96 = 3.92 yd
h = 5/(1.96)² = 1.30 yd
w = 1.96 yd
Explanation:
The volume is given by:

Where:
- l is the long
- w the wide
- h the height
We know that l = 2w, so we have:
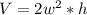
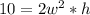
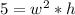 (2)
(2)
Now, the surface of this parallelepiped is:
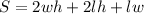
Using l = 2w:

Using (2) we obtain the surface equation in terms of w.
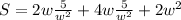
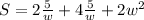
We need to take the derivative with respect to w to minimize the surface area.
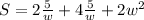
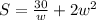
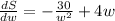
Now, let's equal it to zero.

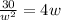

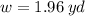
So, l = 2*1.96 = 3.92 yd and h = 5/(1.96)² = 1.30 yd
Therefore, the dimensions are:
l = 2*1.96 = 3.92 yd
h = 5/(1.96)² = 1.30 yd
w = 1.96 yd
I hope it helps you!