Question

Divide.
(4x4 - 4x2 – x-3) = (2x2 – 3)

Answer 1

Given:

Division problem:

`(4x^4-4x^2-x-3)/ (2x^2-3)`

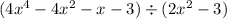
To find:

The solution for the given division problem.

Solution:

We have,

`(4x^4-4x^2-x-3)/ (2x^2-3)`

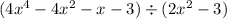
Here, 4x^4-4x^2-x-3 is the dividend and (2x^2-3) is divisor.

Using the long division method we get

`\underline{2x^2-3}|\overline{4x^4-4x^2-x-3}|\underline{2x^2+1}`

`\underline{4x^4-6x^2}`

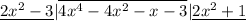
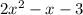`2x^2-x-3` (After subtraction)

`\underline{2x^2\quad \quad -3}`

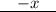`\underline{\quad -x\quad}` (After subtraction)

Now,

`(4x^4-4x^2-x-3)/(2x^2-3)=2x^2+1-(x)/(2x^2-3)`

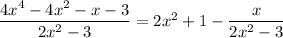
Therefore, the required solution is
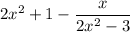`2x^2+1-(x)/(2x^2-3)`.