Given:
The number of tickets = 1000
Mathew buys a single ticket for $8
Winning price = $800
To find:
The expected earning per ticket.
Solution:
It is given that the total number of tickets is 1000. So, the probability of winning is:
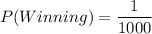
The probability of losing is:
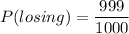
The expected earning per ticket is:
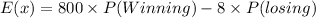
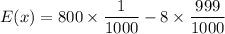
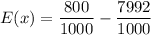
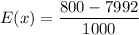
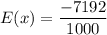
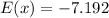
Therefore, the expected earning per ticket is -7.192. Here, negative sign means loss of $7.192.