Question

Write the equation of the line graphed below in Point-Slope form. Use the point labeled on the graph. State the slope and point.

Answer 1

Given:

The graph of a line.

To find:

The point-slope form of the given line.

Solution:

From the given graph it is clear that the line passes through the point (1,5) and (0,-1). So, the slope of the line is:

`m=(y_2-y_1)/(x_2-x_1)`

`m=(-1-5)/(0-1)`

`m=(-6)/(-1)`

`m=6`

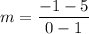
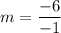
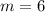
The slope of the line is 6 and it passes through the point (1,5). So, the point slope form of the line is:

`y-y_1=m(x-x_1)`

`y-5=6(x-1)`

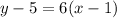
Therefore, the point-slope form is
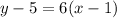`y-5=6(x-1)`, slope is 6 and the point is (1,5).