Question

Given a leading coefficient of 8, polynomial roots of 1 & 2, and the known point on the graph (4,5). Write an equation that will find the

missing 3rd root (r). You do not have to solve the problem. See the example "Find the Missing Root - Interesting Twist" if you need help.


_=_(_-1)(_-2)(_-_)

Answer 1

Given:

The leading coefficient of a polynomial is 8.

Polynomial roots are 1 and 2.

The graph passes through the point (4,5).

To find:

The 3rd root and the equation of the polynomial.

Solution:

The factor form of a polynomial is:

`y=a(x-c_1)(x-c_2)...(x-c_n)`

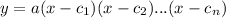
Where, a is a constant and
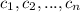`c_1,c_2,...,c_n` are the roots of the polynomial.

Polynomial roots are 1 and 2. So,
`(x-1)` and
`(x-2)` are the factors of the polynomial.

Let the third root of the polynomial by c, then
`(x-c)` is a factor of the polynomial.

The leading coefficient of a polynomial is 8. So, a=8 and the equation of the polynomial is:

`y=8(x-1)(x-2)(x-c)`

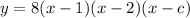
The graph passes through the point (4,5). Putting
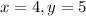`x=4,y=5`, we get

`5=8(4-1)(4-2)(4-c)`

`5=8(3)(2)(4-c)`

`5=48(4-c)`

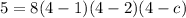
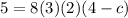
Divide both sides by 48.

`(5)/(48)=4-c`

`c=4-(5)/(48)`

`c=(192-5)/(48)`

`c=(187)/(48)`

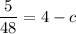
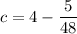
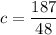
Therefore, the 3rd root on the polynomial is
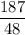`(187)/(48)`.