Answer:
The probability of getting a reading between -1.42 degree C and 1.61 degree C is 0.8685.
Explanation:
We are given that
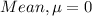 degree C
degree C
Standard deviation,
 degree C
degree C
We have to find the probability of the reading between -1.42 and 1.61.
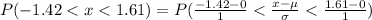
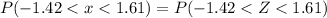

Using the formula
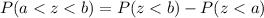

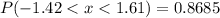
Hence, the probability of getting a reading between -1.42 degree C and 1.61 degree C is 0.8685.