Answer:
b) LeBron is relatively taller because he has a larger z-score.
Explanation:
Z-score:
In a set with mean
 and standard deviation
and standard deviation
 , the z-score of a measure X is given by:
, the z-score of a measure X is given by:

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.
LeBron James:
Height of 81 inches, while the average 30- to 39-year old man is 69.5 inches tall, with a standard deviation of 2.7 inches, which means that we have to find Z when
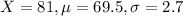



Candace Parker:
Height of 76 inches, while the average 30- to 39-year old woman is 64.2 inches tall, with a standard deviation of 3.2 inches. This means that we have to find Z when
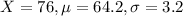

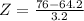
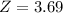
Who is relatively taller?
Due to the higher z-score, LeBron James, and thus, the correct answer is given by option b.