Answer:
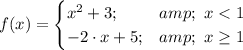
Explanation:
The y-intercept (when x = 0) on the quadratic graph is 3, the upper quadratic graph does not intersect the x-axis, and the endpoint of the function has an open circle that stops at x = 1. The starting point extends to the boundaries of the graph
Therefore, the piecewise function shown in the quadratic graph is given as follows;
f(x) = x² + 3; x < 1
The straight line graph has a negative slope and starts at x = 1, with a closed circle, extending to higher values of x to the boundaries of the graph
Two points on the straight line graph are (1, 3) and (5, -5), therefore, the slope is (-5 - 3)/(5 - 1) = -2
The equation of the function is y - 3 = -2·(x - 1)
∴ y = f(x) -2·x + 2 + 3 = -2·x + 5
f(x) = -2·x + 5; x ≥ 1