Question

How many joules of energy are required to accelerate one kilogram of mass from rest to a velocity of 0.866c?

Answer 1

the amount of energy needed is 1.8 x 10¹⁷ J.

Step-by-step explanation:

Given;

mass of the object, m₀ = 1 kg

velocity of the object, v = 0.866 c

By physics convection, c is the speed of light = 3 x 10⁸ m/s

The energy needed is calculated as follows;

E = Mc²

As the object approaches the speed of light, the change in the mass of the object is given by Einstein's relativity formula;

`M = \frac{M_0}{\sqrt{1- (v^2)/(c^2) } } \\\\ M = \frac{1}{\sqrt{1- ((0.866c)^2)/(c^2) } }\\\\ M = \frac{1}{\sqrt{1- (0.74996c^2)/(c^2) } }\\\\ M = (1)/(√(0.25) ) \\\\ M = 2 \ kg`

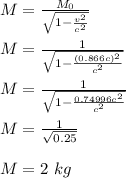
The energy required is calculated as;

E = 2 x (3 x 10⁸)²

E = 1.8 x 10¹⁷ J

Therefore, the amount of energy needed is 1.8 x 10¹⁷ J.