Answer:
The dimensions of the rectangle are 8 by 7 centimeters.
Explanation:
The length of a rectangle is 13 centimeters less than three times its width. In other words:

Given that the area of the rectangle is 56 square centimeters, we want to determine its dimensions.
Recall that the area of a rectangle is given by:
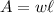
Substitute in known values and equations:
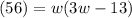
Solve for w. Distribute:

Isolate the equation:

Factor. We want to find two numbers that multiply to 3(-56) = -168 and that add to -13.
-21 and 8 suffice. Hence:
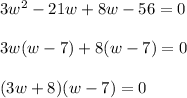
Zero Product Property:
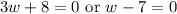
Solve for each case:
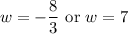
Since the width cannot be negative, we can ignore the first solution.
Therefore, the width of the rectangle is seven centimeters.
Thus, the length will be:

Thus, the dimensions of the rectangle are 8 by 7 centimeters.