Answer:
B.) P(A/B) = P(A)
Explanation:
If two events, A and B are independent:
We have that:
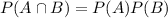
Conditional Probability
We use the conditional probability formula to solve this question. It is

In which
P(B|A) is the probability of event B happening, given that A happened.
 is the probability of both A and B happening.
is the probability of both A and B happening.
P(A) is the probability of A happening.
Since they are independent:
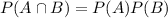
Then

So
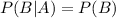 , or either:
, or either:
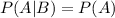 , and thus, the correct answer is given by option B.
, and thus, the correct answer is given by option B.