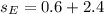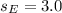Missing from the question
Aletheia's speed is 0.6 miles per hour slower than Elvira's speed.
Answer:
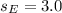
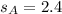
Explanation:
Given
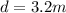 -- distance
-- distance
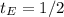 --- Elvira time
--- Elvira time
 --- Aletheia time
--- Aletheia time
 --- the relationship between their speeds
--- the relationship between their speeds
Required
Their walking speed
Distance (d) is calculated as:
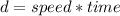
For Elvira, we have:
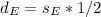
For Aletheia, we have:

So, we have:
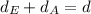 --- total distance
--- total distance
This gives:
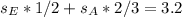
Recall that:

Make sE the subject
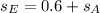
Substitute
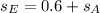 in
in
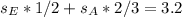
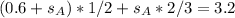
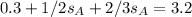
Collect like terms
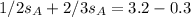
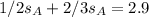
Express all as decimal
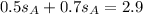
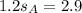
Divide both sides by 1.2
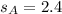
Recall that:
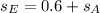
So, we have: