Answer:
The man would be 40 years old.
Explanation:
Blood pressure as function of age:
Is given by the following equation:
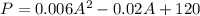
Find the age of a man whose normal blood pressure measures 129 mmHg.
This is A for which P = 129. So
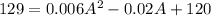

Solving a quadratic equation:
Given a second order polynomial expressed by the following equation:
 .
.
This polynomial has roots
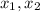 such that
such that
 , given by the following formulas:
, given by the following formulas:
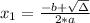

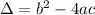
In this question:
Quadratic equation with
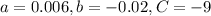 . So
. So
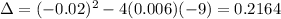
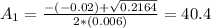
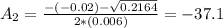
Age has to be a positive number, so rounding to the nearest year:
The man would be 40 years old.