Question

Given the figure, which method will you most

likely use to prove that triangle ADE and
triangle ABC are similar?
A.The SSS Postulate
B.The SAS Postulate
C.The ASA Postulate
D.The AA Postulate

Answer 1

B. The SAS Postulate

Step-by-step explanation:

In the given figure, we are shown two triangles,
`\triangle ADE` and
`\triangle ABC`.

Since triangle ADE is inscribed in triangle ABC, both triangles must share angle
`A`. Furthermore, let's take a look at the two legs of each triangle, if we say that their respective bases are DE and BC.

Compare the corresponding legs of each triangle with proportions:

`(AC)/(AE)=(10)/(5)=2,\\\\(AB)/(AD)=(8)/(4)=2,\\\\\overline{AC}:\overline{AE}=\overline{AB}:\overline{AD}`

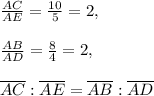
Since two corresponding legs/sides of triangle are in a constant proportion, the triangles must be similar from the SAS (Side-Angle-Side) Postulate.

Answer 2

Answer:
B. The SAS Postulate

Step-by-step explanation:
Both triangles share angle A, and their sides can be proven similar because the ratios of 4:8 and 5:10.