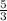Answer:
x =
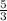
Explanation:
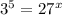
These problems are getting you ready to work with exponential functions,
and ultimately with logarithms. The point here is that with variable exponents (notice the exponent is an x (on the right one) and not a number. Variable exponents can not be solved with regular algebra "rules" you need new ones.
The new ones will be Logs....
For now (until you learn logs) , you have to use some "tricks"
the "trick" in this problem is that you have to realize that 27 =
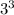 ...
...
with "common bases" this problem becomes trivial
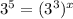
so now the bases are the same and the equals sign suggests that
3x = 5
thus x =