Question

A navy diver hears the underwater sound wave from an exploding ship across the harbor. They immediately lift their head out of the water. The sound wave from the explosion propagating through the air reaches the diver 4.00 s later. The sound velocity is 1440 m/s in water How far away is the ship?

Answer 1

s = 1800 m = 1.8 km

Step-by-step explanation:

The distance, the speed, and the time of reach of the sound are related by the following formula:

`s = vt`

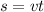
where,

s = distance

v = speed

t = time

FOR WATER:

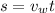`s = v_wt` ---------------------- eq (1)

where,

s = distance between ship and diver = ?

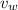`v_w` = speed of sound in water = 1440 m/s

t = time taken by sound in water

FOR AIR:

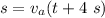`s = v_a(t+4\ s)` ---------------------- eq (2)

where,

s = distance between ship and diver = ?

`v_a` = speed of sound in water = 344 m/s

t + 4 s = time taken by sound in water

Comparing eq (1) and eq (2),because distance remains constant:

`v_wt=v_a(t+4\ s)\\\\(1440\ m/s)t = (344\ m/s)(t+4\ s)\\(1440\ m/s - 344\ m/s)t=1376\ m\\t = (1376\ m)/(1096\ m/s)`

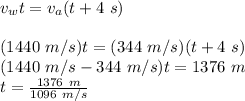
t = 1.25 s

Now using this value in eq (1):

`s = (1440\ m/s)(1.25\ s)`

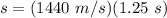
s = 1800 m = 1.8 km