Answer:
6) 6

9) 40
10)

11) 13
Explanation:
6)A right triangle rule: if 2 legs are equal, the hypotenuse is the length of that leg*

9) Pythagorean Theorem
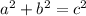
We know the hypotenuse (41) so we substitute that for c and 9 for b now we need to find a
 which gives us 40
which gives us 40
10) same with #6 but we do the opposite. SInce we have the hypotenuse, we can divide that by
 because we know that if 2 legs are equal, the hypotenuse is multiplied by
because we know that if 2 legs are equal, the hypotenuse is multiplied by
 . Multiply the numerator and denominator by
. Multiply the numerator and denominator by
 because we can't have a square root in the denominator.
because we can't have a square root in the denominator.
11) like #9 we have the a and b but we need to find c
a=5 b=12 c=r
so
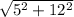 which gives us 13
which gives us 13