Question

An astronaut on the moon throws a baseball upward. the astronaut is 6​ ft, 6 in.​ tall, and the initial velocity of the ball is 50 ft per sec. the height s of the ball in feet is given by the equation s=-2.7t^2+50t+6.5 ​, where t is the number of seconds after the ball was thrown. complete parts a and b.

A) After how many seconds is the ball 10 ft above the​ moon's surface?

B)How many seconds will it take for the ball to hit the​ moon's surface?

Answer 1

Explanation:

The position function is

`s(t)=-2.7t^2+50t+6.5` and if we are looking for the time(s) that the ball is 10 feet above the surface of the moon, we sub in a 10 for s(t) and solve for t:

`10=-2.7t^2+50t+6.5` and

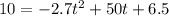`0=-2.7t^2+50t-3.5` and factor that however you are currently factoring quadratics in class to get

t = .07 sec and t = 18.45 sec

There are 2 times that the ball passes 10 feet above the surface of the moon, once going up (.07 sec) and then again coming down (18.45 sec).

For part B, we are looking for the time that the ball lands on the surface of the moon. Set the height equal to 0 because the height of something ON the ground is 0:

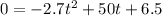`0=-2.7t^2+50t+6.5` and factor that to get

t = -.129 sec and t = 18.65 sec

Since time can NEVER be negative, we know that it takes 18.65 seconds after launch for the ball to land on the surface of the moon.