Question

if sin(θ)=7/25, when π/2<θ<π, use the given information about θ to find the exact value of sin(θ/2)

Answer 1

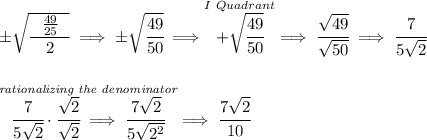
we know that π/2 < θ < π, which is another way of saying that θ is in the II Quadrant, so half that angle will most likely be located on the I Quadrant, where cosine as well as sine are both positive.

Now, let's keep in mind that θ itself is in the II Quadrant, where the cosine is negative whilst the sine is positive.

`\textit{Half-Angle Identities} \\\\ sin\left(\cfrac{\theta}{2}\right)=\pm \sqrt{\cfrac{1-cos(\theta)}{2}} \\\\[-0.35em] \rule{34em}{0.25pt}\\\\ sin(\theta )=\cfrac{\stackrel{opposite}{7}}{\underset{hypotenuse}{25}}\qquad \textit{let's now find the \underline{adjacent side}} \\\\\\ \textit{using the pythagorean theorem} \\\\ c^2=a^2+b^2\implies \pm√(c^2-b^2)=a \qquad \begin{cases} c=hypotenuse\\ a=adjacent\\ b=opposite\\ \end{cases}`

`\pm√(25^2-7^2)=a\implies \pm√(576)=a\implies \pm 24=a\implies \stackrel{II~Quadrant}{-24=a} \\\\[-0.35em] ~\dotfill\\\\ cos(\theta )=\cfrac{\stackrel{adjacent}{-24}}{\underset{hypotenuse}{25}} \\\\\\ sin\left(\cfrac{\theta}{2}\right)\implies \pm \sqrt{\cfrac{1-\left( -(24)/(25) \right)}{2}}\implies \pm \sqrt{\cfrac{1 +(24)/(25) }{2}}`

`\pm\sqrt{\cfrac{~~(49)/(25) ~~}{2}}\implies \pm\sqrt{\cfrac{49}{50}}\implies \stackrel{I~Quadrant}{+\sqrt{\cfrac{49}{50}}}\implies \cfrac{√(49)}{√(50)}\implies \cfrac{7}{5√(2)} \\\\\\ \stackrel{\textit{rationalizing the denominator}}{\cfrac{7}{5√(2)}\cdot \cfrac{√(2)}{√(2)}\implies \cfrac{7√(2)}{5√(2^2)}}\implies \cfrac{7√(2)}{10}`