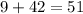Question

What is the area of this polygon

Answer 1

51

Explanation:

1. Approach

One is given the polygon, (ABCDE); the problem asks one to find the area of this polygon. The most logical step to take is to divide this polygon into easier parts, find the area of each part, and then add up the area to find the total area of the figure.

One way to divide this figure up is to draw the line (AC). This will create the triangle (ABC) and rectangle (ACDE).

2. Find the area of (ABC)

The formula to find the area of a triangle is the following:

`A=(b*h)/(2)`

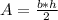
Where (b) is the base of the triangle, and (h) is the height. The base of the triangle (ABC) is (AC), which has a measure of (6) units. The height of the triangle is the distance from the base of the triangle to the vertex opposite the base. This measurement is (3) units. Substitute these values into the formula and solve for the area:

`A=(b*h)/(2)`

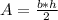
Substitute,

`A=(6*3)/(2)\\\\A=(18)/(2)\\\\A=9`

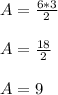
3. Find the area of (ACDE)

The formula to find the area of a rectangle is as follows:

`A=b*h`

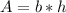
The base of the rectangle is the segment (AE), with a measure of (7) units. The height of the rectangle is the segment (AC) with a measurement of (6) units. Substitute these values into the formula and solve for the area:

`A=7*6\\\\A=42`

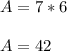
4. Find the area of the total figure

To find the area of the total figure, add up the area of the triangle, and the area of the rectangle:

`9+42= 51`