Question

A survey sampled men and women workers and asked if they expected to get a raise or promotion this year. Suppose the survey sampled 200 men and 200 women. If 98 of the men replied Yes and 72 of the women replied Yes, are the results statistically significant so that you can conclude a greater proportion of men expect to get a raise or a promotion this year?

a. State the hypothesis test in terms of the population proportion of men and the population proportion of women.
b. What is the sample proportion for men? For women?
c. Use α= 0.01 level of significance. What is the p-value and what is your conclusion?

Answer 1

a)

The null hypothesis is:
`H_0: p_M - p_W = 0`

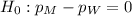
The alternative hypothesis is:
`H_1: p_M - p_W > 0`

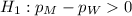
b) For men is of 0.49 and for women is of 0.36.

c) The p-value of the test is 0.0039 < 0.01, which means that the results are statistically significant so that you can conclude a greater proportion of men expect to get a raise or a promotion this year.

Explanation:

Before solving this question, we need to understand the central limit theorem and subtraction of normal variables.

Central Limit Theorem

The Central Limit Theorem establishes that, for a normally distributed random variable X, with mean
`\mu` and standard deviation
`\sigma`, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
`\mu` and standard deviation
`s = (\sigma)/(√(n))`.

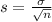For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.

For a proportion p in a sample of size n, the sampling distribution of the sample proportion will be approximately normal with mean
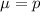`\mu = p` and standard deviation
`s = \sqrt{(p(1-p))/(n)}`

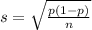
Subtraction between normal variables:

When two normal variables are subtracted, the mean is the difference of the means, while the standard deviation is the square root of the sum of the variances.

Men:

98 out of 200, so:

`p_M = (98)/(200) = 0.49`

`s_M = \sqrt{(0.49*0.51)/(200)} = 0.0353`

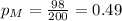
Women:

72 out of 200, so:

`p_W = (72)/(200) = 0.36`

`s_W = \sqrt{(0.36*0.64)/(200)} = 0.0339`

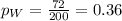
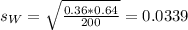
a. State the hypothesis test in terms of the population proportion of men and the population proportion of women.

At the null hypothesis, we test if the proportion are similar, that is, if the subtraction of the proportions is 0, so:

`H_0: p_M - p_W = 0`

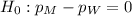
At the alternative hypothesis, we test if the proportion of men is greater, that is, the subtraction is greater than 0, so:

`H_1: p_M - p_W > 0`

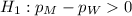
b. What is the sample proportion for men? For women?

For men is of 0.49 and for women is of 0.36.

c. Use α= 0.01 level of significance. What is the p-value and what is your conclusion?

From the sample, we have that:

`X = p_M - p_W = 0.49 - 0.36 = 0.13`

`s = √(s_M^2+s_W^2) = √(0.0353^2 + 0.0339^2) = 0.0489`

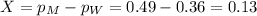
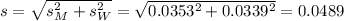
The test statistic is:

`z = (X - \mu)/(s)`

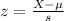
In which X is the sample mean,
`\mu` is the value tested at the null hypothesis, and s is the standard error, so:

`z = (0.13 - 0)/(0.0489)`

`z = 2.66`

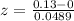
P-value of the test and decision:

The p-value of the test is the probability of finding a difference above 0.13, which is the p-value of z = 2.66.

Looking at the z-table, z = 2.66 has a p-value of 0.9961.

1 - 0.9961 = 0.0039.

The p-value of the test is 0.0039 < 0.01, which means that the results are statistically significant so that you can conclude a greater proportion of men expect to get a raise or a promotion this year.