Question

A fan that is rotating at 960 rev/s is turned off. It makes 1500 revolutions before it comes to a stop. a) What was its angular acceleration(assuming it was constant)

Answer 1

α = 1930.2 rad/s²

Step-by-step explanation:

The angular acceleration can be found by using the third equation of motion:

`2\alpha \theta=\omega_f^2-\omega_i^2`

where,

α = angular acceleration = ?

θ = angular displacement = (1500 rev)(2π rad/1 rev) = 9424.78 rad

ωf = final angular speed = 0 rad/s

ωi = initial angular speed = (960 rev/s)(2π rad/1 rev) = 6031.87 rad/s

Therefore,

`2\alpha(9424.78\ rad) = (0\ rad/s)^2-(6031.87\ rad/s)^2\\\\\alpha = -((6031.87\ rad/s)^2)/((2)(9424.78\ rad))`

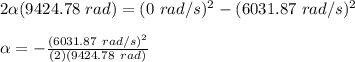
α = - 1930.2 rad/s²

negative sign shows deceleration