Answer:
The 99% confidence interval for the difference in the two proportions is (-0.0247, 0.2833).
Explanation:
Before building the confidence interval, we need to understand the Central Limit Theorem and subtraction of normal variables.
Central Limit Theorem
The Central Limit Theorem establishes that, for a normally distributed random variable X, with mean
 and standard deviation
and standard deviation
 , the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
, the sampling distribution of the sample means with size n can be approximated to a normal distribution with mean
 and standard deviation
and standard deviation
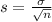 .
.
For a skewed variable, the Central Limit Theorem can also be applied, as long as n is at least 30.
For a proportion p in a sample of size n, the sampling distribution of the sample proportion will be approximately normal with mean
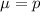 and standard deviation
and standard deviation
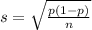
A BYU-Idaho professor took a survey of his classes and found that 82 out of 90 people who had served a mission had personally met a member of the quorum of the twelve apostles.
This means that:
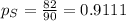
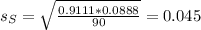
Of the non-returned missionaries that were surveyed 86 of 110 had personally met a member of the quorum of the twelve apostles.
This means that:
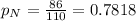
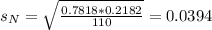
Distribution of the difference:

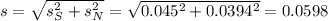
Calculate a 99% confidence interval for the difference in the two proportions.
The confidence interval is:

In which
z is the z-score that has a p-value of
 .
.
99% confidence level
So
 , z is the value of Z that has a p-value of
, z is the value of Z that has a p-value of
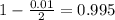 , so
, so
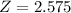 .
.
The lower bound of the interval is:
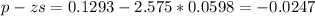
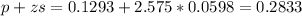
The 99% confidence interval for the difference in the two proportions is (-0.0247, 0.2833).