Answer:
0.0287 = 2.87% probability the total of the 100 claims exceeds 1,530,000.
Explanation:
Normal Probability Distribution
Problems of normal distributions can be solved using the z-score formula.
In a set with mean
 and standard deviation
and standard deviation
 , the z-score of a measure X is given by:
, the z-score of a measure X is given by:

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.
n instances of a normal variable:
For n instances of a normal variable, the mean is
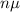 and the standard deviation is
and the standard deviation is
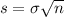
Sum of normal variables:
When two normal variables are added, the mean is the sum of the means, while the standard deviation is the square root of the sum of the variances.
Group A follow a normal distribution with mean 10,000 and standard deviation 1,000. 50 claims of group A.
This means that:
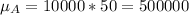
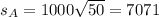
Group B follow a normal distribution with mean 20,000 and standard deviation 2,000. 50 claims of group B.
This means that:
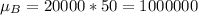
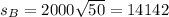
Distribution of the total of the 100 claims:
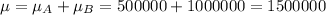
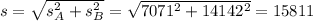
Find the probability the total of the 100 claims exceeds 1,530,000.
This is 1 subtracted by the p-value of Z when X = 1530000. So

By the Central Limit Theorem
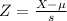

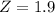
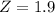 has a p-value of 0.9713
has a p-value of 0.9713
1 - 0.9713 = 0.0287
0.0287 = 2.87% probability the total of the 100 claims exceeds 1,530,000.