Question

In the Fuel Economy Guide for 2018 model vehicles, the Environmental Protection Agency gives data on 1160 vehicles. There are a number of outliers, mainly hybrid gas electric vehicles. If we ignore outliers, the combined city and highway gas mileage of the other 1134 vehicles is approximately Normal with mean 22.6 miles per gallon (mpg) and standard deviation 5.2 mpg.

Required:
a. What proportion of vehicles have worse gas mileage than the 2020 Honda Civic?
b. My dad has a truck that gets around 14 mpg. How many standard deviations from the mean is my dad's truck?

Answer 1

a) The probability is the p-value of
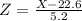`Z = (X - 22.6)/(5.2)`, in which X is the gas mileage of the 2020 Honda Civic.

b) Your dad's truck is 1.65 standard deviations below the mean.

Explanation:

Normal Probability Distribution

Problems of normal distributions can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

Normal with mean 22.6 miles per gallon (mpg) and standard deviation 5.2 mpg.

This means that
`\mu = 22.6, \sigma = 5.2`

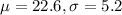
a. What proportion of vehicles have worse gas mileage than the 2020 Honda Civic?

This is the p-value of Z, given by:

`Z = (X - \mu)/(\sigma)`

`Z = (X - 22.6)/(5.2)`

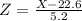
In which X is the gas mileage of the 2020 Honda Civic.

b. My dad has a truck that gets around 14 mpg. How many standard deviations from the mean is my dad's truck?

This is Z when X = 14. So

`Z = (X - \mu)/(\sigma)`

`Z = (14 - 22.6)/(5.2)`

`Z = -1.65`

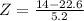
Your dad's truck is 1.65 standard deviations below the mean.