Answer:
The weight that separate the top 8% by 5.2605 and the weight that separate bottom 8% by 5.1195.
Explanation:
We are given that
Mean,
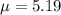
Standard deviation,

We have to find the two weights that separate the top 8% and the bottom 8%.
Let x1 and x2 the two weights that separate the top 8% and the bottom 8%.
Z-value for p-value =0.08 =
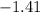
For 8% bottom
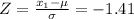
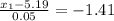


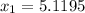
For 8% top
p-Value=1-0.08=0.92
Z- value=1.41
Now,



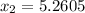
(x1,x2)=(5.1195,5.2605)