Question

Use the coordinates of the labeled point to find the point-slope equation of

the line
-5
5
-5
(3.-5)
O A. y+ 5 = -3(x - 3)
B. y- 5 = -3(x+3)
C. y + 5 = 3(x+3)
O D. Y-3 - (x+5)

Answer 1

A. y + 5 = -3(x - 3)

Step-by-step explanation:

Given the following data;

Points on the graph (x1, y1) = (3, -5)

Points on the graph (x2, y2) = (0, 4)

First of all, we would determine the slope of the equation of line;

Mathematically, the slope of a line is given by the formula;

`Slope, \ m = \frac {Change \; in \; y-axis}{Change \; in \; x-axis}`

`Slope, \ m = \frac {y_(2) - y_(1)}{x_(2) - x_(1)}`

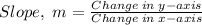
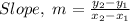
Substituting into the formula, we have;

`Slope, \ m = \frac {4 - (-5)}{0 - 3}`

`Slope, \ m = \frac {4 + 5}{0 - 3}`

`Slope, \ m = \frac {9}{-3}`

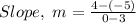
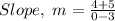
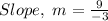
Slope, m = -3

Next, to find the point-slope equation of the line, we would use the following formula;

y - y1 = m(x - x1)

y - (-5) = -3(x - 3)

y + 5 = -3x + 9

y = -3x + 9 - 5

y = -3x + 4 = mx + c