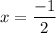Question

Use the quadratic formula to find the solution to the quadratic equation given

below.
X^2-x+1/4=0

Answer 1

`\displaystyle x=(-1)/(2)`

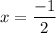
General Formulas and Concepts:

Pre-Algebra

Order of Operations: BPEMDAS

1. Brackets
2. Parenthesis
3. Exponents
4. Multiplication
5. Division
6. Addition
7. Subtraction

• Left to Right

Algebra I

• Standard Form: ax² + bx + c = 0
• Quadratic Formula:
  `\displaystyle x=(-b \pm √(b^2 - 4ac))/(2a)`

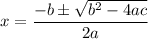
Explanation:

Step 1: Define

Identify

x² + x + 1/4 = 0

↓ Compare to Standard Form

a = 1, b = 1, c = 1/4

Step 2: Solve for x

1. Substitute in variables [Quadratic Formula]:
   `\displaystyle x=\frac{-1 \pm \sqrt{1^2 - 4(1)((1)/(4))}}{2(1)}`
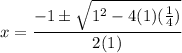
2. [√Radical] Evaluate exponents:
   `\displaystyle x=\frac{-1 \pm \sqrt{1 - 4(1)((1)/(4))}}{2(1)}`
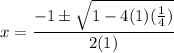
3. [√Radical] Multiply:
   `\displaystyle x=(-1 \pm √(1 - 1))/(2(1))`
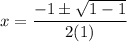
4. [√Radical] Subtract:
   `\displaystyle x=(-1 \pm √(0))/(2(1))`
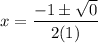
5. [√Radical] Evaluate:
   `\displaystyle x=(-1 \pm 0)/(2(1))`
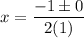
6. Simplify:
   `\displaystyle x=(-1)/(2(1))`
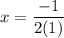
7. Multiply:
   `\displaystyle x=(-1)/(2)`