Question

Help differentiate this

Answer 1

`\displaystyle y' = 20x^3 + 6x^2 + 70x + 9`

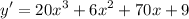
General Formulas and Concepts:

Pre-Algebra

• Distributive Property

Algebra I

• Terms/Coefficients
• Expand by FOIL
• Functions
• Function Notation

Calculus

Derivatives

Derivative Notation

Derivative Property [Multiplied Constant]:
`\displaystyle (d)/(dx) [cf(x)] = c \cdot f'(x)`

Derivative Property [Addition/Subtraction]:
`\displaystyle (d)/(dx)[f(x) + g(x)] = (d)/(dx)[f(x)] + (d)/(dx)[g(x)]`

Basic Power Rule:

1. f(x) = cxⁿ
2. f’(x) = c·nxⁿ⁻¹

Derivative Rule [Product Rule]:
`\displaystyle (d)/(dx) [f(x)g(x)]=f'(x)g(x) + g'(x)f(x)`

Explanation:

Step 1: Define

Identify

`\displaystyle y = (x^3 + 7x - 1)(5x + 2)`

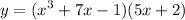
Step 2: Differentiate

1. Product Rule:
   `\displaystyle y' = (d)/(dx)[(x^3 + 7x - 1)](5x + 2) + (x^3 + 7x - 1)(d)/(dx)[(5x + 2)]`
2. Basic Power Rule [Derivative Property - Addition/Subtraction]:
   `\displaystyle y' = (3x^(3 - 1)+ 7x^(1 - 1) - 0)(5x + 2) + (x^3 + 7x - 1)(5x^(1 - 1) + 0)`
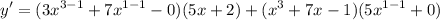
3. Simplify:
   `\displaystyle y' = (3x^2+ 7)(5x + 2) + 5(x^3 + 7x - 1)`
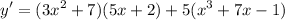
4. Expand:
   `\displaystyle y' = 15x^3 + 6x^2 + 35x + 14 + 5(x^3 + 7x - 1)`
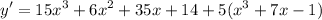
5. [Distributive Property] Distribute 5:
   `\displaystyle y' = 15x^3 + 6x^2 + 35x + 14 + 5x^3 + 35x - 5`
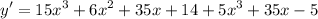
6. Combine like terms:
   `\displaystyle y' = 20x^3 + 6x^2 + 70x + 9`

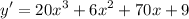
Topic: AP Calculus AB/BC (Calculus I/I + II)

Unit: Derivatives

Book: College Calculus 10e