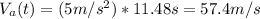Answer:
Let's define t = 0s (the initial time) as the moment when Car A starts moving.
Let's find the movement equations of each car.
A:
We know that Car A accelerations with a constant acceleration of 5m/s^2
Then the acceleration equation is:
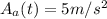
To get the velocity, we integrate over time:

Where V₀ is the initial velocity of Car A, we know that it starts at rest, so V₀ = 0m/s, the velocity equation is then:

To get the position equation we integrate again over time:
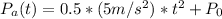
Where P₀ is the initial position of the Car A, we can define P₀ = 0m, then the position equation is:
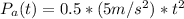
Now let's find the equations for car B.
We know that Car B does not accelerate, then it has a constant velocity given by:
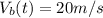
To get the position equation, we can integrate:
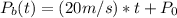
This time P₀ is the initial position of Car B, we know that it starts 100m ahead from car A, then P₀ = 100m, the position equation is:
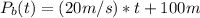
Now we can answer this:
1) The two cars will meet when their position equations are equal, so we must have:
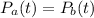
We can solve this for t.
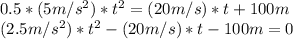
This is a quadratic equation, the solutions are given by the Bhaskara's formula:

We only care for the positive solution, which is:

Car A reaches Car B after 11.48 seconds.
2) How far does car A travel before the two cars meet?
Here we only need to evaluate the position equation for Car A in t = 11.48s:
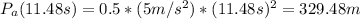
3) What is the velocity of car B when the two cars meet?
Car B is not accelerating, so its velocity does not change, then the velocity of Car B when the two cars meet is 20m/s
4) What is the velocity of car A when the two cars meet?
Here we need to evaluate the velocity equation for Car A at t = 11.48s