Answer:
Step-by-step explanation:
From the information given:
The motional emf can be computed by using the formula:
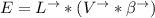
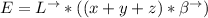
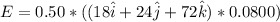
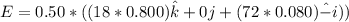

E = 0.72 volts
According to the question, suppose the wire segment was parallel, there will no be any emf induced since the magnetic field is present along the y-axis.
As such, for any motional emf should be induced, the magnetic field, length, and velocity are required to be perpendicular to one another .
Then the motional emf will be:
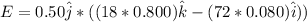
E = 0 (zero)