Question

If the length of a is 6, the length of b is 4, and the length of c is 7, what is the measure of angle B

Answer 1

B = 34.77°

Explanation:

For this problem you are given all three side lengths and asked to find an angle. To do this you can use the law of cosines which is written:

`b^(2) =a^(2) +c^(2) -2ac Cos(B)`

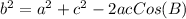
This can be rearranged to find the angle B:

`b^(2) -a^(2) -c^(2) /-2ac = Cos(B)`

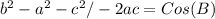
With lowercase letters being sides and uppercase being angles.

We simply plug in the sides and solve:

`4^(2) -6^(2) -7^(2)/-2*6*7= Cos(B)`

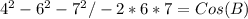
0.8214 = Cos (B)

Then you use inverse cosine to get angle B alone.

B = 34.77°