Answer:
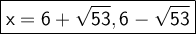
Explanation:
We would like to solve the given quadratic equation by completing the square method .The given equation is ;
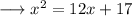
Subtract 12x to both sides ,
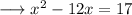
Here the co-efficient of x² is already 1 . So , we shall rewrite it as ,
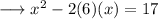
Add 6² on both sides ,
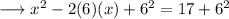
Now LHS is in the form of (a-b)² = a²-2ab+b² ; so that ;
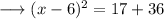
Simplify RHS ,

Put square root on both sides,
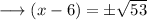
Add 6 on both sides,

Separate the two solutions ,
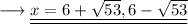
And we are done!