Answer:
There are two alternatives: (i) Polar coordinate system (a.k.a. Circular coordinate system), (ii) Elliptic coordinate system.
Explanation:
There are two alternative ways of describing the location of points on a plane:
(i) Polar coordinate system (a.k.a. Circular coordinate system).
(ii) Elliptic coordinate system.
Now we proceed to explain briefly the characteristic of each option:
Polar coordinate system:
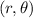
Where:
 - Distance of the point with respect to origin.
- Distance of the point with respect to origin.
 - Direction of the vector between origin and point with respect to the +x semiaxis, in sexagesimal degrees.
- Direction of the vector between origin and point with respect to the +x semiaxis, in sexagesimal degrees.
The formulae for each component in terms of Cartesian coordinates are described below:
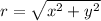 (1)
(1)
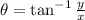 (2)
(2)
Elliptic coordinate system:
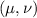
Where
 and
and
 are elliptical coordinates.
are elliptical coordinates.
The formulae for each component in terms of Cartesian coordinates are described below:
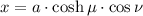 (3)
(3)
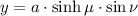 (4)
(4)
Where
 is the distance between origin and any of the foci along the x axis.
is the distance between origin and any of the foci along the x axis.