Question

Hannah would like to make an investment that will turn 8000 dollars into 33000 dollars in 7 years. What quarterly rate of interest, compounded four times per year, must she receive to reach her goal?

Answer 1

20.76%

Explanation:

`33000=8000(1+(i)/(4))^(4*7)\\4.125=(1+(i)/(4))^(28)\\\sqrt[28]{4.125}=1+(i)/(4) \\i= .207648169`

which rounds to 20.76%

Answer 2

About 0.2076 or 20.76%.

Explanation:

Recall that compound interest is given by the formula:

`\displaystyle A=P\left(1+(r)/(n)\right)^(nt)`

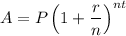
Where A is the final amount, P is the principal, r is the interest rate, n is the number of times the interest is applied per year, and t is the number of years.

Since Hannah wants to turn an $8,000 investment into $33,000 in seven years compounded quarterly, we want to solve for r given that P = 8000, A = 33000, n = 4, and t = 7. Substitute:

`\displaystyle \left(33000\right)=\left(8000\right)\left(1+(r)/(4)\right)^((4)(7))`

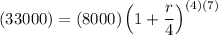
Simplify and divide both sides by 8000:

`\displaystyle (33)/(8)=\left(1+(r)/(4)\right)^(28)`

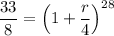
Raise both sides to the 1/28th power:

`\displaystyle \left((33)/(8)\right)^{{}^(1)\! / \! {}_(28)}= 1+(r)/(4)`

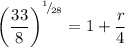
Solve for r. Hence:

`\displaystyle r= 4\left(\left((33)/(8)\right)^{{}^(1)\! / \! {}_(28)}-1\right)`

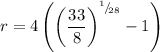
Use a calculator. Hence:

`r=0.2076...\approx 0.2076`

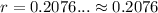
So, the quarterly rate of interest must be 0.2076, or about 20.76%.