Question

Describe the motion of a particle with position (x, y) as t varies in the given interval. (For each answer, enter an ordered pair of the form x, y.) x = 1 + sin(t), y = 3 + 2 cos(t), π/2 ≤ t ≤ 2π

Answer 1

The motion of the particle describes an ellipse.

Explanation:

The characteristics of the motion of the particle is derived by eliminating
`t` in the parametric expressions. Since both expressions are based on trigonometric functions, we proceed to use the following trigonometric identity:

`\cos^(2) t + \sin^(2) t = 1` (1)

Where:

`\cos t = (y-3)/(2)` (2)

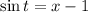`\sin t = x - 1` (3)

By (2) and (3) in (1):

`\left((y-3)/(2) \right)^(2) + (x-1)^(2) = 1`

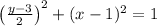
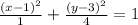`((x-1)^(2))/(1)+((y-3)^(2))/(4) = 1` (4)

The motion of the particle describes an ellipse.