Question

Mathematically verify the outlier(s) in the data set using the 1.5 rule.

7, 8, 11, 13, 14, 14, 14, 15, 16, 16, 18, 19

1. 7 & 19 are outliers.
2. 7 & 8 are outliers.
3. 7, 8, & 19 are outliers.
4. There are no outliers.

Answer 1

Given:

The data values are:

7, 8, 11, 13, 14, 14, 14, 15, 16, 16, 18, 19

To find:

The outliers of the given data set.

Solution:

We have,

7, 8, 11, 13, 14, 14, 14, 15, 16, 16, 18, 19

Divide the data set in two equal parts.

(7, 8, 11, 13, 14, 14), (14, 15, 16, 16, 18, 19)

Divide each parenthesis in two equal parts.

(7, 8, 11), (13, 14, 14), (14, 15, 16), (16, 18, 19)

Now,

`Q_1=(11+13)/(2)`

`Q_1=(24)/(2)`

`Q_1=12`

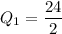
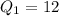
And

`Q_3=(16+16)/(2)`

`Q_3=(32)/(2)`

`Q_3=16`

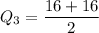
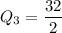
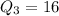
The interquartile range is:

`IQR=Q_3-Q_1`

`IQR=16-12`

`IQR=4`

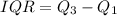
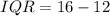
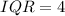
The data values lies outside the interval
`[Q_1-1.5IQR,Q_3+1.5IQR]` are known as outliers.

`[Q_1-1.5IQR,Q_3+1.5IQR]=[12-1.5(4),16+1.5(4)]`

`[Q_1-1.5IQR,Q_3+1.5IQR]=[12-6,16+6]`

`[Q_1-1.5IQR,Q_3+1.5IQR]=[6,22]`

All the data values lie in the interval [6,22]. So, there are no outliers.

Hence, the correct option is 4.