Question

A shell is fired at an initial speed of 2500 m/s at an initial angle of 45 degrees. Find the shell's horizontal range and the amount of time the shell is in motion. (note that because it is fired from the ground to the ground, they displacement = 0.)

Answer 1

d= 637323 meters

t= 360.5 seconds

Answer 2

Step-by-step explanation:

Since the height from which the shell was fired is the same as the height at which it lands (on the ground, to be specific), we will use the range equation. That is the only time you CAN use the range equation (when the initial height and the final height are exactly the same). The range equation is:

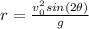`r=(v_0^2sin(2\theta))/(g)` where v0 is the initial velocity, theta is the angle, and g is the pull of gravity (NOT negative). Filling in:

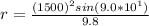`r=((1500)^2sin(9.0*10^1))/(9.8)` so, doing all that math gives us:

r = 6.4 × 10⁵ meters