Question

6. Calculate the area of the octagon in the
figure below.

Answer 1

`41\text{ [units squared]}`

Explanation:

The octagon is irregular, meaning not all sides have equal length. However, we can break it up into other shapes to find the area.

The octagon shown in the figure is a composite figure as it's composed of other shapes. In the octagon, let's break it up into:

• 4 triangles (corners)
• 3 rectangles (one in the middle, two on top after you remove triangles)

Formulas:

• Area of rectangle with length
  `l` and width
  `w`:
  `A=lw`
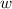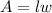
• Area of triangle with base
  `b` and height
  `h`:
  `A=(1)/(2)bh`

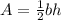
Area of triangles:

All four triangles we broke the octagon into are congruent. Each has a base of 2 and a height of 2.

Thus, the total area of one is
`A=(1)/(2)\cdot 2\cdot 2=2\text{ square units}`

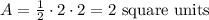
The area of all four is then
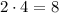`2\cdot 4=8` units squared.

Area of rectangles:

The two smaller rectangles are also congruent. Each has a length of 3 and a width of 2. Therefore, each of them have an area of
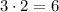`3\cdot 2=6` units squared, and the both of them have a total area of
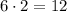`6\cdot 2=12` units squared.

The last rectangle has a width of 7 and a height of 3 for a total area of
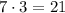`7\cdot 3=21` units squared.

Therefore, the area of the entire octagon is
`8+12+21=\boxed{41\text{ [units squared]}}`