Question

If z = x² + y² , prove that:


`{ \large{ \rm{ {x}^(2) \: {\frac{ {∂}^(2)z }{∂ {x}^(2) } + 2xy \frac{ {∂}^(2)z }{∂x∂y} } + {y}^(2) \frac{ {∂}^(2)z }{∂ {y}^(2) } = 2z}}}`
_____________
Don't Spam
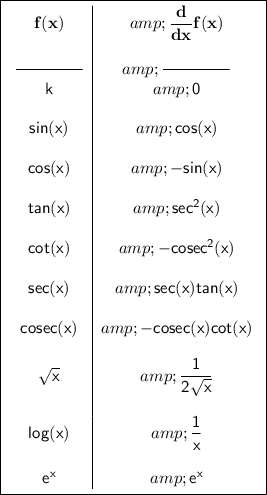
Well explained answers needed
​

Answer 1

Refer to the attachments for answer

Used Concept :-

• 
  `{\boxed{\bf{(d)/(dx)(x^n)=nx^(n-1)}}}`

Answer 2

Given That:

`{ \large\longrightarrow { \rm{z = {x}^(2) + {y}^(2) }}}`

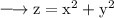
Partial differentiating both sides with respect to x, we get:

`{ \large\longrightarrow { \rm{ (∂z)/(∂x) = 2x}}}`

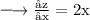
Partial differentiating again with respect to x, we get:

`{ \large\longrightarrow {{ \rm{ \frac{ {∂}^(2)z }{∂ {x}^(2) } \: = 2 \: \: \: \: \quad -(i) }}}}`

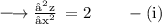
Consider again:

`{ \large\longrightarrow {{ \rm{ z = {x}^(2) + {y}^(2) }}}}`

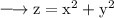
Partial differentiating both sides with respect to y, we get:

`{ \large\longrightarrow { { \rm{ (∂z)/(∂y) = 2y}}}}`

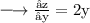
Now, partial differentiating both sides with respect to x, we get:

`{ \large\longrightarrow{ \rm{ \frac{ {∂}^(2)z }{∂x \:∂y } = 0 \: \: \: \quad - (ii) }}}`

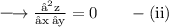
Consider again:

`{ \large\longrightarrow { \rm{ (∂z)/(∂y) = 2y}}}`

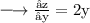
Partial differentiating both sides with respect to y, we get:

`{ \large\longrightarrow{ \rm{ \frac{ {∂}^(2)z }{∂ {y}^(2) } = 2 \: \: \: \: \: \quad - (iii) }}}`

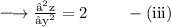
Now, consider Left Hand Side, we get:

`{ \large{ \rm{ \longrightarrow {x}^(2) \frac{ {∂}^(2)z }{∂ {x}^(2) } + 2xy \frac{ {∂}^(2) x}{∂x \:∂y } + {y}^(2) \frac{ {∂}^(2)z }{∂ {y}^(2) } }}}`

From Equation (i),(ii) and (iii), we can write:

`\longrightarrow{ \large{ \rm{ {2x}^(2) + 0 + {2y}^(2) }}}`

`\longrightarrow \: { \large{ \rm{2( {x}^(2) + {y}^(2) ) }}}`

`\longrightarrow{ \large{ \rm{2z}}}`

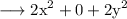
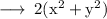
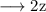
Therefore,

`{ \large{\longrightarrow \: { \rm{{x}^(2) \frac{ {∂}^(2) z}{∂ {x}^(2) } + 2xy \frac{ {∂}^(2)x }{∂x \:∂y } + {y}^(2) \frac{ {∂}^(2)z }{∂ {y}^(2) } = 2z }}}}`

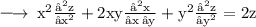
Hence Proved!!

`\:`

Learn More:-

`\boxed{\begin{array}c\bf f(x)&\bf(d)/(dx)f(x)\\ \\ \frac{\qquad\qquad}{}&\frac{\qquad\qquad}{}\\ \sf k&\sf0\\ \\ \sf sin(x)&\sf cos(x)\\ \\ \sf cos(x)&\sf-sin(x)\\ \\ \sf tan(x)&\sf{sec}^(2)(x)\\ \\ \sf cot(x)&\sf-{cosec}^(2)(x)\\ \\ \sf sec(x)&\sf sec(x)tan(x)\\ \\ \sf cosec(x)&\sf-cosec(x)cot(x)\\ \\ \sf√(x)&\sf(1)/(2√(x))\\ \\ \sf log(x)&\sf(1)/(x)\\ \\ \sf{e}^(x)&\sf{e}^(x)\end{array}}`