Question

Solve for y


`2y - 3 = \sqrt{ {3y}^(2) - 10y + 12}`
absurd answers will be reported!!​

Answer 1

y = 3

Explanation:

2y - 3 =
`√(3y^2-10y+12)`

square both sides to remove sqrt bracket

(2y - 3)^2 = (
`√(3y^2-10y+12)` )^2

simplify both sides

(2y - 3)(2y - 3) =
`3y^2` - 10y + 12

`4y^2` - 12y + 9 =
`3y^2` - 10y + 12

bring all value to left side

`y^2` - 2y - 3 = 0

factor

(y - 3)(y + 1)

solve for y

y = 3, y = -1

When plugged back into the equation, only y = 3 is true

Answer 2

y = 3

Step-by-step explanation :

`2y - 3 = √( 3y² - 10y + 12)`

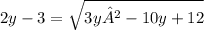
Swap the sides both of the equation.

`√( 3y² - 10y + 12) = 2y - 3`

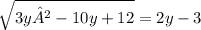
To remove the brackets of equations square both side and simplify .

3y² - 10y + 12 = 4y² - 12y + 9

Move the expression to left-hand side and change its sign.

3y² - 10y + 12 - 4y² + 12y - 9 = 0

collect like terms

3y² - 4y² - 10y + 12y + 12 - 9 = 0

-y² + 2y + 3 = 0

Change the sign of expression. because it helps to solve.

y² - 2y - 3 = 0

Splits the term -2y

y² + y -3y - 3 = 0

Factor out y from the first pair and -3 from second pair of expression.

y ( y + 1 ) - 3 ( y + 1) = 0

Factor out y + 1 from the expression.

( y + 1 ) ( y - 3 ). = 0

When product and factors equals 0. at least one factor is 0.

y + 1 =0

y - 3 = 0

Solve for y

y = -1 and y = 3

If we plug the 3 as y in the expression we find that y = 3 is the true solution of this expression.

This equation has one solution which is y = 3.