9514 1404 393
Answer:
1/3
Explanation:
The slope of a line is the ratio of its "rise" to its "run." The "rise" is the change in vertical distance, and the "run" is the corresponding change in horizontal distance between two points on the line. The formula for the slope is ...

In this problem, you are told the line passes through the origin, which is point (x, y) = (0, 0), and through point (6, 2). Using these coordinates in the slope formula gives ...
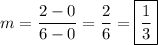
__
You may notice that when the line passes through the origin, the slope is simply the ratio y/x of any point on the line. Here, that ratio is 2/6 = 1/3.
_____
Additional comment
A line through the origin is the graph of a proportional relationship. That is, y is proportional to x. The slope of the line is the constant of proportionality. The equation of the line is ...
y = kx . . . . . . where k is the constant of proportionality.
The line in this problem statement will have the equation ...
y = (1/3)x