Answer:
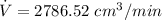
Step-by-step explanation:
Given:
initial pressure during adiabatic expansion of air,
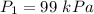
initial volume during the process,
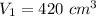
The adiabatic process is governed by the relation
 ; where C is a constant.
; where C is a constant.
Rate of decrease in pressure,
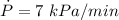
Then the rate of change in volume,
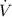 can be determined as:
can be determined as:
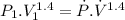
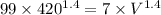
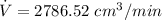
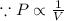
 The rate of change in volume will be increasing.
The rate of change in volume will be increasing.