Question

Two methods, A and B, are available for teaching Spanish. There is a 70% chance of successfully learning Spanish if method A is used, and a 85% chance of success if method B is used. However, method B is substantially more time consuming and is therefore used only 20% of the time (method A is used the other 80% of the time). The following notations are suggested:

A—Method A is used.
B—Method B is used.
L—Spanish was learned successfully. A person learned Spanish successfully.

What is the probability that he was taught by method A?

Answer 1

0.7671 = 76.71% probability that he was taught by method A

Explanation:

Conditional Probability

We use the conditional probability formula to solve this question. It is

`P(B|A) = (P(A \cap B))/(P(A))`

In which

P(B|A) is the probability of event B happening, given that A happened.

`P(A \cap B)` is the probability of both A and B happening.

P(A) is the probability of A happening.

In this question:

Event A: Person learned Spanish successfully.

Event B: Method A was used.

Probability of a person learning Spanish successfully:

70% of 80%(using method A)

85% of 20%(using method B)

So

`P(A) = 0.7*0.8 + 0.85*0.2 = 0.73`

Probability of a person learning Spanish successfully and using method A:

70% of 80%, so:

`P(A \cap B) = 0.7*0.8 = 0.56`

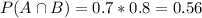
What is the probability that he was taught by method A?

`P(B|A) = (P(A \cap B))/(P(A)) = (0.56)/(0.73) = 0.7671`

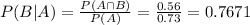
0.7671 = 76.71% probability that he was taught by method A