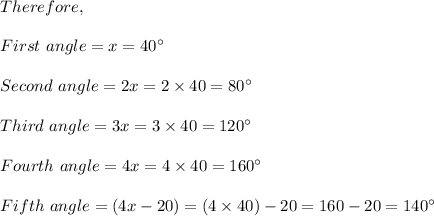Answer:

Explanation:
Let the first angle be = x
Given:
Second angle is twice first = 2x
Third angle is three times first = 3x
Also given:
Second angle is half of fourth angle, that is:

Fifth angle is 20° less than fourth angle, that is:

Sum of interior angles of a polygon with n sides = ( n - 2 ) x 180°
Here n = 5 ,
therefore sum of interior angles = ( 5 - 2 ) x 180 = 3 x 180 = 540°.
That is ,
x + 2x + 3x + 4x + ( 4x - 20 ) = 540
14x - 20 = 540
14x = 540 + 20
14x = 560
x = 40