Answer:
2 ( Option A )
Explanation:
The given integral to us is ,
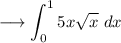
Here 5 is a constant so it can come out . So that,

Now we can write √x as ,
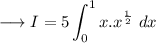
Simplify ,
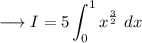
By Power rule , the integral of x^3/2 wrt x is , 2/5x^5/2 . Therefore ,
![\longrightarrow I = 5 \bigg( (2)/(5) x^{(5)/(2)} \bigg] ^1_0 \bigg)](https://img.qammunity.org/2022/formulas/mathematics/high-school/2w5xjb4hhnr9kvvmrp0e52ax0cz4vn9yz6.png)
On simplifying we will get ,
