Question

In an English test, the mean is 60 and the standard deviation is 6. Assuming the scores are normally distributed, answer the following question.

a. what is the percentile rank of the score 65?
b. what is the percentile of the score less than 70?
c. what is percent of the scores is greater than 65?
d. what percent of scores is less than 70?
e. what percent of the score is between 50 and 60?

Answer 1

a) A score of 65 is in the 79.67th percentile.

b) A score less than 70 is below the 95.25th percentile.

c) 20.33% of the scores are greater than 65.

d) 95.25% of scores are less than 70.

e) 45.25% of the scores are between 50 and 60.

Explanation:

Normal Probability Distribution

Problems of normal distributions can be solved using the z-score formula.

In a set with mean
`\mu` and standard deviation
`\sigma`, the z-score of a measure X is given by:

`Z = (X - \mu)/(\sigma)`

The Z-score measures how many standard deviations the measure is from the mean. After finding the Z-score, we look at the z-score table and find the p-value associated with this z-score. This p-value is the probability that the value of the measure is smaller than X, that is, the percentile of X. Subtracting 1 by the p-value, we get the probability that the value of the measure is greater than X.

The mean is 60 and the standard deviation is 6.

This means that
`\mu = 60, \sigma = 6`

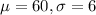
a. what is the percentile rank of the score 65?

This is the p-value of Z when X = 65.

`Z = (X - \mu)/(\sigma)`

`Z = (65 - 60)/(6)`

`Z = 0.83`

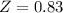
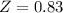`Z = 0.83` has a p-value of 0.7967.

Thus: A score of 65 is in the 79.67th percentile.

b. what is the percentile of the score less than 70?

Below the p-value of Z when X = 70. So

`Z = (X - \mu)/(\sigma)`

`Z = (70 - 60)/(6)`

`Z = 1.67`

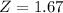
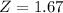`Z = 1.67` has a p-value of 0.9525.

Thus: A score less than 70 is below the 95.25th percentile.

c. what is percent of the scores is greater than 65?

The proportion is 1 subtracted by the p-value of Z when X = 65.

From item a, when X = 65, Z has a p-value of 0.7967

1 - 0.7967 = 0.2033

0.2033*100% = 20.33%

20.33% of the scores are greater than 65.

d. what percent of scores is less than 70?

The proportion is the p-value of Z when X = 70, which, from item b, is of 0.9525.

0.9525*100% = 95.25%

95.25% of scores are less than 70.

e. what percent of the score is between 50 and 60?

The proportion is the p-value of Z when X = 60 subtracted by the p-value of Z when X = 50.

X = 60

`Z = (X - \mu)/(\sigma)`

`Z = (60 - 60)/(6)`

`Z = 0`

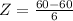
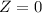
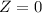`Z = 0` has a p-value of 0.5.

X = 50

`Z = (X - \mu)/(\sigma)`

`Z = (50 - 60)/(6)`

`Z = -1.67`

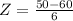
`Z = -1.67` has a p-value of 0.0475.

0.5 - 0.0475 = 0.4525

0.4525*100% = 45.25%

45.25% of the scores are between 50 and 60.